Yield to maturity is a theoretical value, which calculates the return on a bond investment assuming that all payments can be reinvested at the same rate as the original bond purchase. For interest bearing notes, yield to maturity cannot be solved algebraically and is calculated using a financial calculator or computer program.
David’s $9,000 bond investment has a yield to maturity of 27.1%. This rate assumes that he can invest the bond interest payment from the first year at the yield to maturity. By the end of the second year, David would collect $14,542 from his original $9,000 investment. This is the same amount of money that would result from two years of 27.1% compounded interest.
Had David bought the bond for $8,000 his bond would have a yield to maturity of 35.6%. This rate assumes that he can invest the bond interest payment from the first year at the yield to maturity. By the end of the second year, David would collect $14,712 from his original $9,000 investment. This is the same amount of money that would result from two years of 35.6% compounded interest.
Payments Received from a 4 year Bond as Compared with a Bank Deposit
|
Time (Years) |
Principal Payment |
Bond Interest |
Reinvested Coupon Interest |
|
1 |
|
$2,000 |
|
|
2 |
$10,000 |
$2,000 |
$712 |
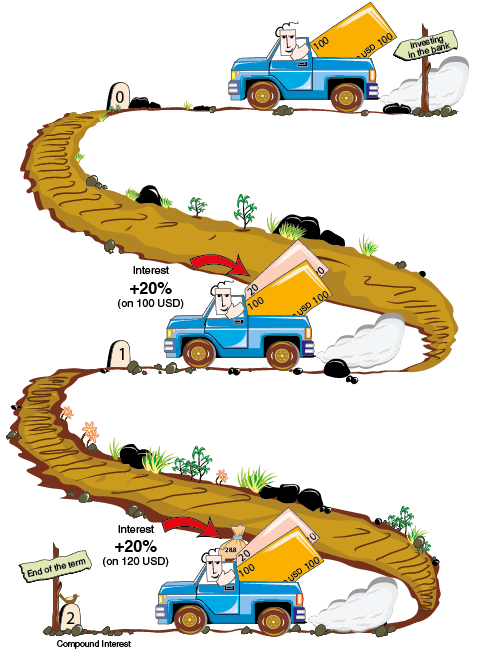
The annual yield to maturity changes according to the amount paid for the bonds
The following example is designed to illustrate this concept more clearly.
Assume that David paid $8,000 for 10 bond certificates, which mature in four years each with a principal value of $1,000. The bonds have a stated interest rate of 20%, which means they pay a $2,000 combined annual coupon.
The following table displays the payments David would receive from principal repayments, bond coupons, and the interest earned by reinvesting the coupons at the bond’s yield to maturity. These payments are listed alongside what he would have earned from investing $8,000 in a savings account with a compound interest rate equal to the yield to maturity (29.1%) of the bond.
This Calculation is Inaccurate
At the end of each year, David can deposit the $2,000 in interest that he receives in the bank, and he will receive annual interest payments, as well.
At the end of four years he will therefore have more than $1,800. The correct annual yield to maturity is consequently approximately 22.6%, not 22.5%.


