What the saver and the debtor have in common is that both acted according to the same decision making formula. Under this formula, if they are offered a sum of money according to the following two alternatives:
-
Alternative 1: Receiving a sum of money today.
-
Alternative 2: Receiving a sum of money at some future date.
So, they will choose the alternative that gives them the greater present value. In other words, they choose the alternative by which they receive the greater amount.
We will demonstrate this through two scenarios: A and B.
Scenario A:

We will choose alternative 1 under scenario A, since the present value of alternative 1 is $1,000 and the present value of alternative 2 is only $800 (remember that the present value of alternative 1 is the sum of money that you can receive today, while the present value of alternative 2 is only $800).
Scenario B:
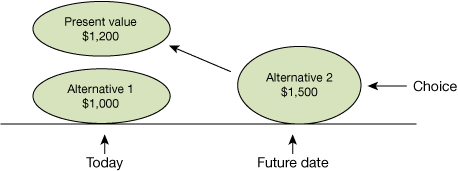
We will choose alternative 2 under scenario B, since the present value of alternative 2 is $1,200, which is greater than the $1,000 present value of alternative 1.
In the previous example, both the saver and the debtor had to decide between receiving a sum of money today and receiving a sum of money in the future. Sometimes, however, we must make decisions about alternatives relating to payments of money, either today or at some future date. We will define the concepts of income and payment more rigorously:
-
Income – an amount of money received today, or at some future date.
- Payment – an amount of money we must pay today, or at some future date.
Choice Between Alternatives in the Case of Payment
Consider the following example:
A large marketer of a given model of television set allows customers to pay according to two alternatives:
- Alternative 1: Payment of $5,000 today.
- Alternative 2: Payment of $5,500 one year from now.
John (wealthy) and Ryan (poor) want to buy television sets.
John’s Considerations:
John has large amounts of money in his current account, which he can deposit in a savings account that yields 8% annual interest. He calculated, and found that in order to pay $5,500 under alternative 2, he must transfer $5,093 today from his current account to a savings plan that will allow them to grow to $5,500 one year from now.
For him, $5,093 is the present value of $5,500 (as of one year from now)
All he has to do, however, is to pay the television set supplier $5,000 today from his current account in order to buy a television set under alternative 1. The obvious conclusion for him is to choose alternative 1.
Ryan’s Considerations:
Ryan is a factory employee, and he has no savings. One year from now, he is due to receive a $5,500 bonus. If he wants to pay today, he must take out a loan bearing 16% annual interest. He can cover the loan from his bonus. Ryan is considering which alternative he should choose.
If he chooses alternative 1, he will take out a $5,000 loan today, which will grow to $5,800 one year from now. The bonus he receives will not be enough to cover the loan. He will therefore choose alternative 2.
Ryan would be indifferent to the choice of alternatives if he had to pay only $4,741 today under alternative 1, since the loan would then grow to only $5,500, which he would pay using his bonus money.
For him, $4,741 is the present value of $5,500 (as of one year from now).
Were the payment required under alternative 1 to be less than $4,741, he would prefer that option, since a loan of less than $4,741 would increase to a sum less than $5,500 one year from now, and he would have money left over from his bonus after repaying the loan that he had received to buy the television set.
The Decision-Making Format: When You Have to Pay
We choose the alternative with the smaller present value (the opposite of the scenario in which we receive money). In other words, we choose the alternative by which we pay the smaller amount of money. We will demonstrate this through two scenarios:
Scenario A involves John, and scenario B involves Ryan.
Scenario A (John):
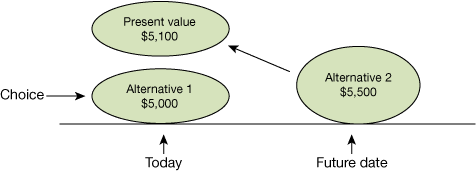
Under scenario A, we will choose alternative 1.
Scenario B (Ryan):
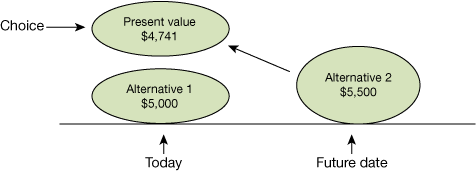
Under scenario A, we will choose alternative 2.
Useful Terms
| Capitalization | The accounting operation for calculating the present value |
| Capitalization Interest | The interest rate used to calculate the present value. We chose 10% for the saver and 20% for the borrower. |
| Amount of Capitalization | A synonym for present value. |
| Flow of Income | A sequence of instalment payments to be received on various dates in the future: for example, $100 every year, for 20 years. |
| Flow of Payments | A sequence of payments of money on various dates in the future: for example, $100 to the bank every year, for 20 years. |


